Part 1: Can Rolling Resistance & Rider Weight be Assumed Constant?

---

In real-time aerodynamic drag and coefficient of drag calculations, it is important to have a good estimate of the rider weight, the rolling resistance coefficient, the power input to the pedals, the wind velocity, and environmental factors. For instance, when selecting a power meter, consumers have an expectation of ‘good’ accuracy. What is not clear, is the quantitative definition of ‘good’ for aerodynamic calculations. What magnitude of error in rider weight and/or rolling resistance would be deemed acceptable in computing aerodynamic drag? This is the focus of the current article, with power meters, wind, and environmental factors coming in subsequent posts.

To answer this question, we will look at real-world experimental data collected using the AeroLab Tech sensor system. Before jumping into the details, let’s first provide some adequate definitions of rider weight and rolling resistance.

---

Rider Weight: the sum of the weight of the bicycle, weight of the person with all equipment carried or held, weight of any filled or unfilled water bottles affixed to the bicycle, and weight of any other auxiliary items (e.g., sensors, bento box).

Rolling Resistance: the resistive force resultant from the rotation of a wheel on a surface. A primitive, but adequate model for the rolling resistance that is presently used by industry as well as the scientific and research community is:

It is important to note that the normal force equation shown above assumes that the bicycle and rider are never producing any aerodynamic downforce or lift (which is observed more commonly in automobile racing dynamics). Now that we have some expressed definitions of Rolling Resistance and Rider Weight, we can consider the equation describing the balance of forces acting on a moving (accelerating) bicycle on an arbitrary road slope with an arbitrary wind and wind angle in the bicycle frame of reference. This may be a bit of an overwhelming equation to start with, but it is elegantly simple to understand if you consider what each term represents in terms of power. A given input power (you pushing on the pedals) is meant to move you forward at a velocity V. Unfortunately, you often experience heavy winds, road slope, and must overcome rolling resistance. Hence, the velocity V varies as you experience these changes. If we consider a (very) short time interval, ∆t, we can state the following mathematical balance which occurs:

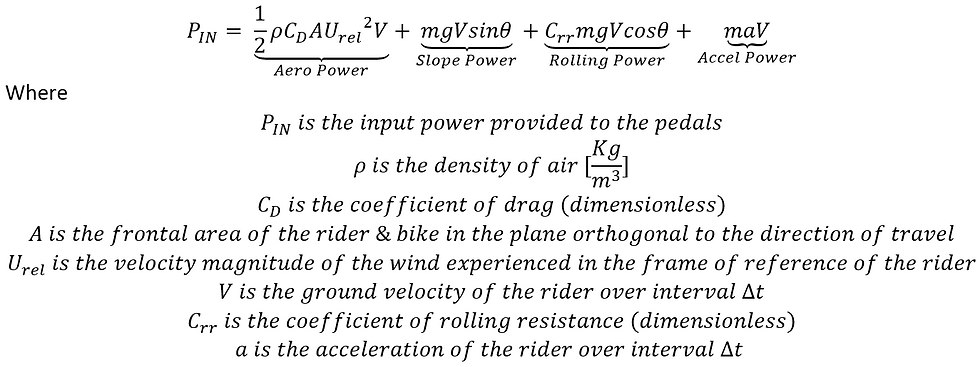
All the variables aside, in the above equation I have highlighted four key terms:

(i) Aero Power – the power to overcome drag,

(ii) Slope Power – the power to overcome the road slope,

(iii) Rolling Power – the power to overcome the rolling resistance, and

(iv) Accel Power – the power to accelerate.

The cumulative sum of these terms ought to balance with the input power over a given short time interval. Interestingly, if one is riding with AeroLab Tech Sensors, most of the variables in this equation can be measured nearly instantaneously. I have intentionally excluded a few additional terms in this equation for brevity, though if a reader is interested, please see the pioneering work by Martin et al. (Martin JC, Milliken DL, Cobb JE, McFadden KL, Coggan AR, “Validation of a mathematical model for road cycling power,” Journal of Applied Biomechanics 14, pp. 276-291, 1998). The purpose of introducing this equation is to have you reflect on where the mass of the rider comes into play, and where the rolling resistance comes into play. We will consider ride data collected using AeroLab Tech sensors, and then methodically introduce errors into the rider mass as well as the rolling resistance. How these errors propagate into estimations of CdA will be the focus.

If you are still reading this and understand the equations presented, congratulations! You are a bike tech nerd like me! (you should consider applying to join our beta testing group!) The remainder of this article is coming soon. The remainder of this article has arrived. Please see Part 2!